Integration By Parts
By DarthVader
Date: 2023-02-25
Topic: 184 see comments
Post views: 892
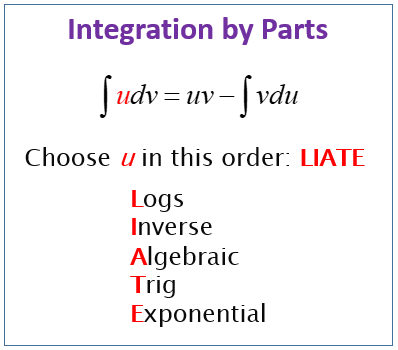
Integration By Parts
Example function: ∫ xe2x dx
To integrate a function such as this, you have to use integration by parts.
Step 1: [ Define “u” ]
Here we will make “u” equal to “x”. E.G. ( u = x )
Step 2: [ Define “dv” ]
Here we will make everything left over equal to “dv”. E.G. ( dv = e2x dx )
Step 3: [ Find “du” ]
Since here “u” is equal to “x”, then: ( du/dx = 1 )
So: (( du = dx )).
Step 4: [ Find “v” ]
Since “dv = e2x dx”, we must integrate both sides to get “v” on its own:
( ∫ dv = ∫ e2x dx ) which equals: ( v = ½ e2x )
Step 5: [ Now use these values with the integration by parts formula to solve the problem ]
∫ u × dv = u × v − ∫ v × du
Note:
The values you need to find are:
“u”
“du”
“v”
“dv”
| Comments | Creator | Date | ID |
|---|